Таким образом, уравнения равновесия для фермы примут вид
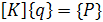 . (11)
. (11)
Решение системы уравнений (8) сводится к вычислению вектора-столбца неизвестных перемещений 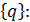
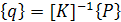 . (12)
. (12)
Существуют стандартные программы решения системы линейных алгебраических уравнений методом Гаусса без нахождения обратной матрицы к матрице жесткости (SIMQ “Fortrun”).
После решения уравнений (11) и нахождения узловых обобщенных перемещений по выражению (1) определяются узловые перемещения в локальной системе координат для каждого элемента
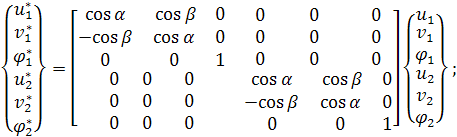 (13)
(13)
По узловым обобщенным перемещениям можно найти величину относительной деформации, соответствующие нормальной силе 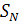 , поперечной силе
, поперечной силе  и изгибающему моменту
и изгибающему моменту  (рис. 4):
(рис. 4):
Рис. 4. Вычисление относительных деформаций
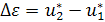 ;
;
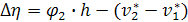 ;
;
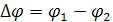 ;
;
Данные соотношения позволяют найти внутренние силы в первом узле:
 , (14)
, (14)
где квадратная матрица есть часть локальной матрицы жесткости (левый верхний угол). Столбцы этой матрицы показывают внутренние силы в первом узле при единичном смещении в соответствующем направлении данного узла и жестком закреплении второго узла. Значения двух силовых факторов постоянны по длине элемента 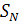 ,
,  и равны в первом и втором узлах элемента.
и равны в первом и втором узлах элемента.
В соответствии с правилом знаков, по которому при положительном изгибающем моменте растянуты нижние волокна
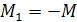
Изгибающий момент во втором узле определим из соотношения:
 ,
,
и линейного характера изменения изгибающего момент в пределах элемента:
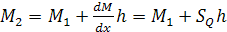 (15)
(15)
На эпюре моментов ось направлена вниз относительно оси элемента 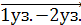
Для решения динамической задачи формируется система уравнений вида:
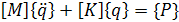 , (16)
, (16)
в которой глобальная матрица масс 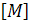 формируется аналогично матрице жесткости путем сложения матриц масс элементов.
формируется аналогично матрице жесткости путем сложения матриц масс элементов.
|
|
Поиск частного решения уравнения (16), при гармоническом внешнем воздействии  , сводится к заданию выражения для обобщенных перемещений
, сводится к заданию выражения для обобщенных перемещений 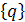 в виде функций, подобных правой части уравнения.
в виде функций, подобных правой части уравнения.
Пусть внешние силы изменяются по закону:
 =
= 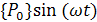 , (17)
, (17)
тогда произвольное частное решение уравнения (16), соответствующее установившемуся режиму представим в виде
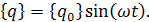 (18)
(18)
Вторая производная от выражения (18) равна
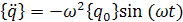
Подставляя выражение (15) в уравнение (13), получим
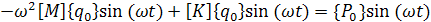
Приравнивая коэффициенты в левой и правой части при 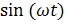 , приходим к линейной системе алгебраических уравнений:
, приходим к линейной системе алгебраических уравнений:
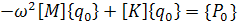 ,
,
вынесем за скобки неизвестные амплитудные значения
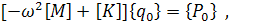 (19)
(19)
Обозначим
 ,
,
тогда уравнение (19) становится аналогичным (11)
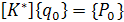 (20)
(20)
В матрице 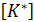 и столбце
и столбце 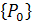 необходимо преобразовать строки, соответствующие перемещениям на которые наложены ограничения (необходимо учесть главные граничные условия), также как это производилось для матрицы [K] при статических расчетах. После система уравнений (20) решается с помощью программы, реализующей метод Гаусса, и находятся амплитуды вынужденных колебаний
необходимо преобразовать строки, соответствующие перемещениям на которые наложены ограничения (необходимо учесть главные граничные условия), также как это производилось для матрицы [K] при статических расчетах. После система уравнений (20) решается с помощью программы, реализующей метод Гаусса, и находятся амплитуды вынужденных колебаний  в решении (18) для установившегося режима.
в решении (18) для установившегося режима.
По амплитудам  , аналогично статическому решению вычисляют амплитуды внутренних силовых факторов по соотношениям (13), (14), (15).
, аналогично статическому решению вычисляют амплитуды внутренних силовых факторов по соотношениям (13), (14), (15).
Текст клиентского кода программы и результаты расчетов в статике:
Форма1
System.Collections.Generic;
using System.ComponentModel;
using System.Data;
using System.Drawing;
using System.Linq;
using System.Text;
using System.Windows.Forms;
using System.IO;
using System.Drawing.Drawing2D;
namespace Рама
{
public partial class Form1: Form
{
public Form2 f2;
Form3 f3;
|
|
List<string> list_st = new List<string>();
string st;
string[] St;
public bool dinamika = false;
public Double[,] MatrCoor, MatrTop;
public double[] q;
public int i = 0, j = 0, n = 0, m = 0;
public Form1()
{
InitializeComponent();
}
private void button1_Click(object sender, EventArgs e)
{
Close();
}
private void сохранитьВФайлToolStripMenuItem_Click(object sender, EventArgs e)
{
//Сохранение в файл координатной матрицы
n = 0;
for (i = 0; i < dataGridView1.RowCount - 1; i++) n++;
MatrCoor = new double[n, 9];
for (i = 0; i < n; i++)
{
for (j = 0; j < 9; j++)
MatrCoor[i, j] = Convert.ToDouble(dataGridView1.Rows[i].Cells[j].Value);
}
if (saveFileDialog1.ShowDialog() == DialogResult.OK)
{
FileStream FS = saveFileDialog1.OpenFile() as FileStream;
StreamWriter SR = new StreamWriter(FS);
//dataGridView1.Rows.Add();
for (i = 0; i < n; i++)
{
st = null;
for (j = 0; j < 9; j++)
{
st = st + MatrCoor[i, j].ToString() + " ";
}
SR.WriteLine(st);
}
SR.Close();
FS.Close();
}
}
private void считатьИзФайлаToolStripMenuItem_Click(object sender, EventArgs e)
{
// Считывание из файла координатной матрицы
if (openFileDialog1.ShowDialog() == DialogResult.OK)
{
FileStream FSR = openFileDialog1.OpenFile() as FileStream;
StreamReader SRR = new StreamReader(FSR);
dataGridView1.Rows.Clear();
list_st.Clear();
while (SRR.Peek() > 0)
{
list_st.Add(SRR.ReadLine());
//dataGridView1
dataGridView1.Rows.Add();
}
St = list_st.ToArray();
n = St.Length;
MatrCoor = new double[n, 9];
// MatrCoor = new Double[n, 7];
for (i = 0; i < n; i++)
{
St[i] = St[i].Replace(".", ",");;
//Разбиение строки St на подстроки aa
string[] aa = St[i].Split(" \t".ToCharArray(), StringSplitOptions.RemoveEmptyEntries);
for (j = 0; j < aa.Length; j++)
{
//Заполнение матрицы и таблицы
MatrCoor[i, j] = Convert.ToDouble(aa[j]);
dataGridView1.Rows[i].Cells[j].Value = MatrCoor[i, j];
}
}
SRR.Close();
FSR.Close();
}
}
private void сохранитьВФайлToolStripMenuItem1_Click(object sender, EventArgs e)
{
// Сохранение в файл топологической матрицы
m = 0;
for (i = 0; i < dataGridView2.RowCount - 1; i++) m++;
MatrTop = new double[m, 11];
for (i = 0; i < m; i++)
{
for (j = 0; j < 10; j++)
MatrTop[i, j] = Convert.ToDouble(dataGridView2.Rows[i].Cells[j].Value);
|
|
}
if (saveFileDialog1.ShowDialog() == DialogResult.OK)
{
FileStream FS = saveFileDialog1.OpenFile() as FileStream;
StreamWriter SR = new StreamWriter(FS);
//dataGridView1.Rows.Add();
for (i = 0; i < m; i++)
{
st = null;
for (j = 0; j < 10; j++)
{
st = st + MatrTop[i, j].ToString() + " ";
}
SR.WriteLine(st);
}
SR.Close();
FS.Close();
}
}
private void считатьИзФайлаToolStripMenuItem1_Click(object sender, EventArgs e)
{
// Считывание из файла топологической матрицы
if (openFileDialog1.ShowDialog() == DialogResult.OK)
{
FileStream FSR = openFileDialog1.OpenFile() as FileStream;
StreamReader SRR = new StreamReader(FSR);
dataGridView2.Rows.Clear();
list_st.Clear();
while (SRR.Peek() > 0)
{
list_st.Add(SRR.ReadLine());
//dataGridView1
dataGridView2.Rows.Add();
}
St = list_st.ToArray();
m = St.Length;
MatrTop = new double[m, 10];
// MatrTop = new Double[m, 10];
for (i = 0; i < m; i++)
{
St[i] = St[i].Replace(".", ",");;
//Разбиение строки St на подстроки aa
string[] aa = St[i].Split(" \t".ToCharArray(), StringSplitOptions.RemoveEmptyEntries);
for (j = 0; j < aa.Length; j++)
{
//Заполнение матрицы и таблицы
MatrTop[i, j] = Convert.ToDouble(aa[j]);
dataGridView2.Rows[i].Cells[j].Value = MatrTop[i, j];
}
}
SRR.Close();
FSR.Close();
}
}
private void button2_Click(object sender, EventArgs e)
{
//Построение
double[] x, y;
Double My = 0, MaxY = 0, Mx = 0, MaxX = 0;
int[] yint, xint;
Bitmap myBmp;
x = new double[n];
y = new double[n];
for (i = 0; i < n; i++)
{
x[i] = MatrCoor[i, 1];
y[i] = MatrCoor[i, 2];
}
if (x == null || y == null) return;
MaxY = 0.001;
MaxX = 0.001;
for (i = 0; i < n; i++)
{
if (System.Math.Abs(y[i]) > MaxY)
{
MaxY = System.Math.Abs(y[i]);;
}
if (System.Math.Abs(x[i]) > MaxX)
{
MaxX = System.Math.Abs(x[i]);
}
}
if (MaxY >= MaxX)
MaxX = MaxY;
else
MaxY = MaxX;
My = (pictureBox1.Height) / 1.2 / MaxY;
Mx = (pictureBox1.Width) / 1.2 / MaxX;
yint = new int[n];
xint = new int[n];
for (int i = 0; i < n; i++)
{
yint[i] = Convert.ToInt32(pictureBox1.Height / 1.1) - Convert.ToInt32(y[i] * My);
xint[i] = Convert.ToInt32(pictureBox1.Width / 10) + Convert.ToInt32(x[i] * Mx);
}
myBmp = new Bitmap(pictureBox1.Width, pictureBox1.Height);
Graphics gr1 = Graphics.FromImage(myBmp);
Pen P1 = new Pen(Color.Red, 2);
gr1.DrawRectangle(P1, 1, 1, pictureBox1.Width - 2, pictureBox1.Height - 2);
Pen P2 = new Pen(Color.Green, 2);
Pen P5 = new Pen(Color.Green, 12);
Brush B2 = new SolidBrush(Color.Azure);
Brush B3 = new SolidBrush(Color.Brown);
int[] Zakx = new int[n];
int[] Zaky = new int[n];
int[] ZakFi = new int[n];
for (i = 0; i < n; i++)
{
Zakx[i] = Convert.ToInt32(MatrCoor[i, 6]);
Zaky[i] = Convert.ToInt32(MatrCoor[i, 7]);
ZakFi[i] = Convert.ToInt32(MatrCoor[i, 8]);
}
Pen P3 = new Pen(Color.Brown, 4);
Pen P6 = new Pen(Color.Aqua, 18);
for (i = 0; i < m; i++)
{
//Построение распределенной нагрузки
if ((MatrTop[i, 3]!= 0) || (MatrTop[i, 4]!= 0) || (MatrTop[i, 5]!= 0) || (MatrTop[i, 6]!= 0))
{
gr1.DrawLine(P6, xint[(int)MatrTop[i, 1] - 1], yint[(int)MatrTop[i, 1] - 1], xint[(int)MatrTop[i, 2] - 1], yint[(int)MatrTop[i, 2] - 1]);
}
//Построение линий конечных элементов
gr1.DrawLine(P3, xint[(int)MatrTop[i, 1] - 1], yint[(int)MatrTop[i, 1] - 1], xint[(int)MatrTop[i, 2] - 1], yint[(int)MatrTop[i, 2] - 1]);
}
//Нанесение прямоугольников на расположение узлов
for (i = 0; i < n; i++)
{
gr1.FillRectangle(B3, xint[i] - 4, yint[i] - 4, 8,8);
gr1.DrawRectangle(P2, xint[i] - 4, yint[i] - 4, 8, 8);
}
// Линии шириной 12 п. в точках закрепления
for (i = 0; i < n; i++)
{
if (Zakx[i] < 0 || Zaky[i] < 0)
{
gr1.DrawLine(P5, xint[i] - 24, yint[i] + 10, xint[i] + 24, yint[i] + 10);
}
//Нанесение окружностей на расположение шарниров
if ((Zakx[i]!= 0 || Zaky[i]!= 0) & (ZakFi[i] == 0))
{
gr1.FillEllipse(B2, xint[i] - 6, yint[i] - 6, 12, 12);
gr1.DrawEllipse(P2, xint[i] - 6, yint[i] - 6, 12, 12);
}
}
double Mp = 0, MaxP = 0.001, P = 0;
double[] Px = new double[n];
double[] Py = new double[n];
double[] PM = new double[n];
int[] intPx = new int[n];
int[] intPy = new int[n];
Pen P4 = new Pen(Color.Indigo, 6);
// Стиль пера с наконечниками
P4.SetLineCap(LineCap.Flat, LineCap.ArrowAnchor, DashCap.Flat);
//Изображение сил и моментов
for (i = 0; i < n; i++)
{
Px[i] = MatrCoor[i, 3];
Py[i] = MatrCoor[i, 4];
PM[i] = MatrCoor[i, 5];
P = Math.Sqrt(Px[i] * Px[i] + Py[i] * Py[i]);
if (MaxP <= P)
MaxP = P;
}
Mp = pictureBox1.Width / 8 / MaxP;
for (i = 0; i < n; i++)
{
intPy[i] = Convert.ToInt32(Py[i] * Mp);
intPx[i] = Convert.ToInt32(Px[i] * Mp);
}
for (i = 0; i < n; i++)
{
if (intPx[i]!= 0 || intPy[i]!= 0)
{
gr1.DrawLine(P4, xint[i], yint[i], xint[i] + intPx[i], yint[i] - intPy[i]);
}
if (PM[i]>0)
{
gr1.DrawArc(P4, xint[i]-20, yint[i]-20, 40,40,0,-150);
}
if (PM[i] < 0)
{
gr1.DrawArc(P4, xint[i] - 20, yint[i] - 20, 40, 40, 0, 150);
}
}
//---------------------------------------------------------------------------------------
//Построение второго слоя после вычисления перемещений
double[] x1 = new double[n];
double[] y1 = new double[n];
double Md = 1;
// Условие продолжения построений
if (q!= null)
{
Md = Convert.ToDouble(textBox2.Text);
for (i = 0; i < 3 * n; i++)
{
if (i % 3 == 0)
{
x1[i / 3] = x[i / 3] + q[i] * Md;
y1[i / 3] = y[i / 3] + q[i+1] * Md;
}
}
int[] yint1 = new int[n];
int[] xint1 = new int[n];
for (int i = 0; i < n; i++)
{
yint1[i] = Convert.ToInt32(pictureBox1.Height / 1.1) - Convert.ToInt32(y1[i] * My);
xint1[i] = Convert.ToInt32(pictureBox1.Width / 10) + Convert.ToInt32(x1[i] * Mx);
}
Pen P11 = new Pen(Color.Aqua, 1);
gr1.DrawRectangle(P11, 1, 1, pictureBox1.Width - 2, pictureBox1.Height - 2);
Pen P12 = new Pen(Color.Green, 1);
Pen P15 = new Pen(Color.Green, 2);
Brush B12 = new SolidBrush(Color.Azure);
Pen P26 = new Pen(Color.Aqua, 9);
Pen P13 = new Pen(Color.Red, 2);
for (i = 0; i < m; i++)
{
if ((MatrTop[i, 3]!= 0) || (MatrTop[i, 4]!= 0) || (MatrTop[i, 5]!= 0) || (MatrTop[i, 6]!= 0))
{
gr1.DrawLine(P26, xint1[(int)MatrTop[i, 1] - 1], yint1[(int)MatrTop[i, 1] - 1], xint1[(int)MatrTop[i, 2] - 1], yint1[(int)MatrTop[i, 2] - 1]);
}
gr1.DrawLine(P13, xint1[(int)MatrTop[i, 1] - 1], yint1[(int)MatrTop[i, 1] - 1], xint1[(int)MatrTop[i, 2] - 1], yint1[(int)MatrTop[i, 2] - 1]);
}
for (i = 0; i < n; i++)
{
// gr1.FillRectangle(B2, xint1[i] - 4, yint1[i] - 4, 8, 8);
gr1.DrawRectangle(P2, xint1[i] - 4, yint1[i] - 4, 8, 8);
}
for (i = 0; i < n; i++)
{
if (Zakx[i] < 0 || Zaky[i] < 0)
{
gr1.DrawLine(P5, xint[i] - 24, yint[i] + 10, xint[i] + 24, yint[i] + 10);
}
if ((Zakx[i]!= 0 || Zaky[i]!= 0) & (ZakFi[i] == 0))
{
gr1.FillEllipse(B2, xint1[i] - 6, yint1[i] - 6, 12, 12);
gr1.DrawEllipse(P2, xint1[i] - 6, yint1[i] - 6, 12, 12);
}
}
Pen P14 = new Pen(Color.Blue, 3);
// Стиль пера с наконечниками
P14.SetLineCap(LineCap.Flat, LineCap.ArrowAnchor, DashCap.Flat);
for (i = 0; i < n; i++)
{
intPy[i] = Convert.ToInt32(Py[i] * Mp);
intPx[i] = Convert.ToInt32(Px[i] * Mp);
}
Pen P24 = new Pen(Color.Blue, 1);
P24.SetLineCap(LineCap.Flat, LineCap.ArrowAnchor, DashCap.Flat);
for (i = 0; i < n; i++)
{
if (intPx[i]!= 0 || intPy[i]!= 0)
{
gr1.DrawLine(P14, xint1[i], yint1[i], xint1[i] + intPx[i], yint1[i] - intPy[i]);
}
if (PM[i] > 0)
{
gr1.DrawArc(P24, xint1[i] - 20, yint1[i] - 20, 40, 40, 0, -150);
}
if (PM[i] < 0)
{
gr1.DrawArc(P24, xint1[i] - 20, yint1[i] - 20, 40, 40, 0, 150);
}
}
}
pictureBox1.Image = myBmp;
}
private void матрицаЖесткостиToolStripMenuItem_Click(object sender, EventArgs e)
{
// Запуск статических вычислений
// Вычисление
// Создание формы 2
f2 = new Form2(this);
f2.Show();
}
private void продольнаяСилаToolStripMenuItem_Click(object sender, EventArgs e)
{
f3 = new Form3(this);
f3.Show();
}
private void матрицаЖесткостиToolStripMenuItem1_Click(object sender, EventArgs e)
{
dinamika = true;
матрицаЖесткостиToolStripMenuItem_Click(sender, e);
dinamika = false;
}
private void амплитудыМоментовToolStripMenuItem_Click(object sender, EventArgs e)
{
продольнаяСилаToolStripMenuItem_Click(sender, e);
}
}
}
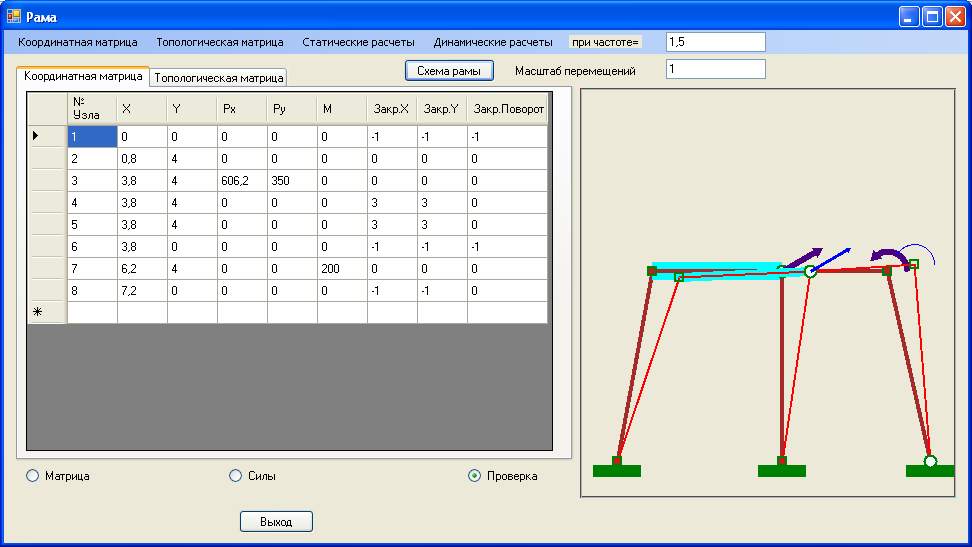
Рис. 5. Главная форма проекта – форма 1
Форма2
using System;
using System.Collections.Generic;
using System.ComponentModel;
using System.Data;
using System.Drawing;
using System.Linq;
using System.Text;
using System.Windows.Forms;
namespace Рама
{
public partial class Form2: Form
{
//Вычисление матрицы жесткости
Form1 f1;
public double[,] K, K1, Ke, Ke1, T, Tt, TtK, M, Me, Me1, TtM, pxy, Qpxy;
public double[] P, P1;
public int[,] A;
public double w = 0;
public Form2(Form1 f1)
{
InitializeComponent();
this.f1 = f1;
Demo();
}
void Demo()
{
if (f1.n > 0)
{
for (int i = 0; i < 3 * f1.n; i++)
{ // Добавление колонки
DataGridViewTextBoxColumn titleColumn1 = new DataGridViewTextBoxColumn();
titleColumn1.HeaderText = "Titlt" + (i + 1).ToString();
//Выравнивание ширины колонки в соответствии с заданным стилем - AllCells
titleColumn1.AutoSizeMode = DataGridViewAutoSizeColumnMode.AllCells;
dataGridView1.Columns.Add(titleColumn1);
dataGridView1.Rows.Add();
}
}
P = new double[3 * f1.n];
P1 = new double[3 * f1.n];
K = new double[3 * f1.n, 3 * f1.n];
M = new double[3 * f1.n, 3 * f1.n];
K1 = new double[3 * f1.n, 3 * f1.n];
A = new int[f1.m, 7];
pxy = new double[f1.m, 4];
Qpxy = new double[f1.m, 6];
double[,] Ht = new double[6, 4];
double[] Qp = new double[6];
for (int i = 0; i < f1.n * 3; i++)
{
if (i % 3 == 0)
{
P[i] = f1.MatrCoor[i / 3, 3];
P[i + 1] = f1.MatrCoor[i / 3, 4];
P[i + 2] = f1.MatrCoor[i / 3, 5];
}
//dataGridView1.Rows[i].Cells[0].Value = P[i];
}
for (int i = 0; i < f1.m; i++)
{
// Формирование матрицы индексов
A[i, 0] = i + 1;
A[i, 1] = Convert.ToInt32(f1.MatrTop[i, 1]) * 3 - 2;
A[i, 2] = Convert.ToInt32(f1.MatrTop[i, 1]) * 3 - 1;
A[i, 3] = Convert.ToInt32(f1.MatrTop[i, 1]) * 3;
A[i, 4] = Convert.ToInt32(f1.MatrTop[i, 2]) * 3 - 2;
A[i, 5] = Convert.ToInt32(f1.MatrTop[i, 2]) * 3 - 1;
A[i, 6] = Convert.ToInt32(f1.MatrTop[i, 2]) * 3;
pxy[i, 0] = f1.MatrTop[i, 3];
pxy[i, 1] = f1.MatrTop[i, 4];
pxy[i, 2] = f1.MatrTop[i, 5];
pxy[i, 3] = f1.MatrTop[i, 6];
}
//Формирование матрицы жесткости и масс
if (f1.dinamika)
w = Convert.ToDouble(f1.textBox1.Text);
for (int ii = 0; ii < f1.m; ii++)
{
Ke = new double[6, 6];
Ke1 = new double[6, 6];
Me = new double[6, 6];
Me1 = new double[6, 6];
T = new double[6, 6];
Tt = new double[6, 6];
TtK = new double[6, 6];
TtM = new double[6, 6];
double x1 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 1]) - 1, 1];
double y1 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 1]) - 1, 2];
double x2 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 2]) - 1, 1];
double y2 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 2]) - 1, 2];
double h = Math.Sqrt((x2 - x1) * (x2 - x1) + (y2 - y1) * (y2 - y1));
double cosA = (x2 - x1) / h;
double cosB = (y2 - y1) / h;
double EF = f1.MatrTop[ii, 7];
double EI = f1.MatrTop[ii, 8];
double mm = f1.MatrTop[ii, 9];
//Матрица преобразования координат
T[0, 0] = cosA;
T[0, 1] = cosB;
T[0, 2] = 0;
T[0, 3] = 0;
T[0, 4] = 0;
T[0, 5] = 0;
T[1, 0] = -cosB;
T[1, 1] = cosA;
T[1, 2] = 0;
T[1, 3] = 0;
T[1, 4] = 0;
T[1, 5] = 0;
T[2, 0] = 0;
T[2, 1] = 0;
T[2, 2] = 1;
T[2, 3] = 0;
T[2, 4] = 0;
T[2, 5] = 0;
T[3, 0] = 0;
T[3, 1] = 0;
T[3, 2] = 0;
T[3, 3] = cosA;
T[3, 4] = cosB;
T[3, 5] = 0;
T[4, 0] = 0;
T[4, 1] = 0;
T[4, 2] = 0;
T[4, 3] = -cosB;
T[4, 4] = cosA;
T[4, 5] = 0;
T[5, 0] = 0;
T[5, 1] = 0;
T[5, 2] = 0;
T[5, 3] = 0;
T[5, 4] = 0;
T[5, 5] = 1;
//Локальная матрица жесткости
Ke[0, 0] = EF / h;
Ke[0, 1] = 0;
Ke[0, 2] = 0;
Ke[0, 3] = -EF / h;
Ke[0, 4] = 0;
Ke[0, 5] = 0;
Ke[1, 0] = 0;
Ke[1, 1] = 12 * EI / Math.Pow(h, 3);
Ke[1, 2] = 6 * EI / Math.Pow(h, 2);
Ke[1, 3] = 0;
Ke[1, 4] = -12 * EI / Math.Pow(h, 3);
Ke[1, 5] = 6 * EI / Math.Pow(h, 2);
Ke[2, 0] = 0;
Ke[2, 1] = 6 * EI / Math.Pow(h, 2);
Ke[2, 2] = 4 * EI / Math.Pow(h, 1);
Ke[2, 3] = 0;
Ke[2, 4] = -6 * EI / Math.Pow(h, 2);
Ke[2, 5] = 2 * EI / Math.Pow(h, 1);
Ke[3, 0] = -EF / h;
Ke[3, 1] = 0;
Ke[3, 2] = 0;
Ke[3, 3] = EF / h;
Ke[3, 4] = 0;
Ke[3, 5] = 0;
Ke[4, 0] = 0;
Ke[4, 1] = -12 * EI / Math.Pow(h, 3);
Ke[4, 2] = -6 * EI / Math.Pow(h, 2);
Ke[4, 3] = 0;
Ke[4, 4] = 12 * EI / Math.Pow(h, 3);
Ke[4, 5] = -6 * EI / Math.Pow(h, 2);
Ke[5, 0] = 0;
Ke[5, 1] = 6 * EI / Math.Pow(h, 2);
Ke[5, 2] = 2 * EI / Math.Pow(h, 1);
Ke[5, 3] = 0;
Ke[5, 4] = -6 * EI / Math.Pow(h, 2);
Ke[5, 5] = 4 * EI / Math.Pow(h, 1);
// Формирование локальной матрицы масс
Me[0, 0] = h / 3;
Me[0, 1] = 0;
Me[0, 2] = 0;
Me[0, 3] = h / 6;
Me[0, 4] = 0;
Me[0, 5] = 0;
Me[1, 0] = 0;
Me[1, 1] = 13.0 / 35 * h;
Me[1, 2] = 11.0 / 210 * h * h;
Me[1, 3] = 0;
Me[1, 4] = 9.0 / 70 * h;
Me[1, 5] = -13.0 / 420 * h * h;
Me[2, 0] = 0;
Me[2, 1] = 9.0 / 70 * h;
Me[2, 2] = 1.0 / 105 * h * h * h;
Me[2, 3] = 0;
Me[2, 4] = 13.0 / 420 * h * h;
Me[2, 5] = -1.0 / 140 * h * h * h;
Me[3, 0] = h / 6;
Me[3, 1] = 0;
Me[3, 2] = 0;
Me[3, 3] = h / 3;
Me[3, 4] = 0;
Me[3, 5] = 0;
Me[4, 0] = 0;
Me[4, 1] = 9.0 / 70 * h;
Me[4, 2] = 13.0 / 420 * h * h;
Me[4, 3] = 0;
Me[4, 4] = 13.0 / 35 * h;
Me[4, 5] = -11.0 / 210 * h * h;
Me[5, 0] = 0;
Me[5, 1] = -13.0 / 420 * h * h;
Me[5, 2] = -1.0 / 140 * h * h * h;
Me[5, 3] = 0;
Me[5, 4] = -11.0 / 210 * h * h;
Me[5, 5] = 1.0 / 105 * h * h * h;
for (int i = 0; i < 6; i++)
for (int j = 0; j < 6; j++)
Me[i, j] = Me[i, j] * mm;
// Матрица для приведения распределенных сил к узловым
Ht[0, 0] = h / 3;
Ht[0, 1] = 0;
Ht[0, 2] = h / 6;
Ht[0, 3] = 0;
Ht[1, 0] = 0;
Ht[1, 1] = 7.0 / 20 * h;
Ht[1, 2] = 0;
Ht[1, 3] = 3.0 / 20 * h;
Ht[2, 0] = 0;
Ht[2, 1] = 1.0 / 20 * h * h;
Ht[2, 2] = 0;
Ht[2, 3] = 1.0 / 30 * h * h;
Ht[3, 0] = h / 6;
Ht[3, 1] = 0;
Ht[3, 2] = h / 3;
Ht[3, 3] = 0;
Ht[4, 0] = 0;
Ht[4, 1] = 7.0 / 20 * h;
Ht[4, 2] = 0;
Ht[4, 3] = 3.0 / 20 * h;
Ht[5, 0] = 0;
Ht[5, 1] = -1.0 / 20 * h * h;
Ht[5, 2] = 0;
Ht[5, 3] = -1.0 / 30 * h * h;
// Формирование вектор столбца сил в локальной системе элемента
for (int j = 0; j < 6; j++)
{
Qp[j] = 0;
for (int k = 0; k < 4; k++)
Qp[j] = Qp[j] + Ht[j, k] * pxy[ii, k];
}
//Преобразование матриц в глобальную систему
for (int i = 0; i < 6; i++)
for (int j = 0; j < 6; j++)
Tt[j, i] = T[i, j];
for (int i = 0; i < 6; i++)
{
for (int j = 0; j < 6; j++)
{
TtK[i, j] = 0;
TtM[i, j] = 0;
for (int k = 0; k < 6; k++)
{
TtK[i, j] = TtK[i, j] + Tt[i, k] * Ke[k, j];
TtM[i, j] = TtM[i, j] + Tt[i, k] * Me[k, j];
}
}
}
for (int i = 0; i < 6; i++)
{
for (int j = 0; j < 6; j++)
{
Ke1[i, j] = 0;
Me1[i, j] = 0;
for (int k = 0; k < 6; k++)
{
Ke1[i, j] = Ke1[i, j] + TtK[i, k] * T[k, j];
Me1[i, j] = Me1[i, j] + TtM[i, k] * T[k, j];
}
}
}
for (int j = 0; j < 6; j++)
{
Qpxy[ii, j] = 0;
for (int k = 0; k < 6; k++)
{
Qpxy[ii, j] = Qpxy[ii, j] + Tt[j, k] * Qp[k];
}
}
for (int i = 1; i <= 6; i++)
{
int ig = A[ii, i];
P[ig - 1] = P[ig - 1] + Qpxy[ii, i - 1];
for (int j = 1; j <= 6; j++)
{
int jg = A[ii, j];
K[ig - 1, jg - 1] = K[ig - 1, jg - 1] + Ke1[i - 1, j - 1];
M[ig - 1, jg - 1] = M[ig - 1, jg - 1] + Me1[i - 1, j - 1];
}
}
}
//Копирование матрицы жесткости в K1
for (int i = 0; i < f1.n * 3; i++)
for (int j = 0; j < f1.n * 3; j++)
{
K1[i, j] = K[i, j];
// dataGridView1.Rows[i].Cells[j].Value = K1[i, j];
}
//Добавление динамической составляющей
for (int i = 0; i < f1.n * 3; i++)
for (int j = 0; j < f1.n * 3; j++)
{
K1[i, j] = K[i, j] - w * w * M[i, j];
//dataGridView1.Rows[i].Cells[j].Value = K1[i, j];
}
for (int i = 0; i < f1.n; i++)
{
//Наложение главных граничных условий для подвижных соединений
if (Convert.ToInt32(f1.MatrCoor[i, 6]) > 0)
{
int sh = Convert.ToInt32(f1.MatrCoor[i, 6]) - 1;
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * sh, j] = K1[3 * sh, j] + K1[3 * i, j];
K1[3 * i, j] = 0;
}
P[3 * sh] = P[3 * i] + P[3 * sh];
P[3 * i] = 0;
K1[3 * i, 3 * sh] = -1.0;
K1[3 * i, 3 * i] = 1.0;
}
if (Convert.ToInt32(f1.MatrCoor[i, 7]) > 0)
{
int sh = Convert.ToInt32(f1.MatrCoor[i, 7]) - 1;
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * sh + 1, j] = K1[3 * sh + 1, j] + K1[3 * i + 1, j];
K1[3 * i + 1, j] = 0;
}
P[3 * sh + 1] = P[3 * i + 1] + P[3 * sh + 1];
P[3 * i + 1] = 0;
K1[3 * i + 1, 3 * sh + 1] = -1.0;
K1[3 * i + 1, 3 * i + 1] = 1.0;
}
if (Convert.ToInt32(f1.MatrCoor[i, 8]) > 0)
{
int sh = Convert.ToInt32(f1.MatrCoor[i, 8]) - 1;
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * sh + 2, j] = K1[3 * sh + 2, j] + K1[3 * i + 2, j];
K1[3 * i + 2, j] = 0;
}
P[3 * sh + 2] = P[3 * i + 2] + P[3 * sh + 2];
P[3 * i + 2] = 0;
K1[3 * i + 2, 3 * sh + 2] = -1.0;
K1[3 * i + 2, 3 * i + 2] = 1.0;
}
}
for (int i = 0; i < f1.n; i++)
{
//Наложение главных граничных условий для неподвижных опор
if (Convert.ToInt32(f1.MatrCoor[i, 6]) < 0)
{
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * i, j] = 0;
}
K1[3 * i, 3 * i] = K[3 * i, 3 * i];
P[3 * i] = 0;
}
if (Convert.ToInt32(f1.MatrCoor[i, 7]) < 0)
{
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * i + 1, j] = 0;
}
K1[3 * i + 1, 3 * i + 1] = K[3 * i + 1, 3 * i + 1];
P[3 * i + 1] = 0;
}
if (Convert.ToInt32(f1.MatrCoor[i, 8]) < 0)
{
for (int j = 0; j < f1.n * 3; j++)
{
K1[3 * i + 2, j] = 0;
}
K1[3 * i + 2, 3 * i + 2] = K[3 * i + 2, 3 * i + 2];
P[3 * i + 2] = 0;
}
}
for (int i = 0; i < f1.n * 3; i++)
{
P1[i] = P[i];
}
int KS = 0;
ClassSIMQ S = new ClassSIMQ();
S.SIMQ(K1, ref P1, f1.n * 3, ref KS);
label2.Text = "Код ошибки равен " + KS.ToString();
f1.q = new double[f1.n * 3];
f1.q = P1;
//Реакции и узловые внешние силы
double[] D = new double[f1.n * 3];
for (int i = 0; i < f1.n * 3; i++)
{
D[i] = 0;
for (int j = 0; j < f1.n * 3; j++)
{
D[i] = D[i] + K[i, j] * f1.q[j];
}
}
//Проверка
double[] D1 = new double[f1.n * 3];
for (int i = 0; i < f1.n * 3; i++)
{
D1[i] = -P[i];
for (int j = 0; j < f1.n * 3; j++)
{
D1[i] = D1[i] + K1[i, j] * f1.q[j];
}
}
int signal = 0;
if (f1.radioButton1.Checked) signal = 1;
if (f1.radioButton2.Checked) signal = 2;
if (f1.radioButton3.Checked) signal = 3;
switch (signal)
{
case 1:
//Вывод матрицы жесткости в таблицу
for (int i = 0; i < f1.n * 3; i++)
for (int j = 0; j < f1.n * 3; j++)
{
dataGridView1.Rows[i].Cells[j].Value = K1[i, j];
}
label1.Text = " Матрица жесткости";
break;
case 2:
for (int i = 0; i < f1.n * 3; i++)
{
dataGridView1.Rows[i].Cells[1].Value = P1[i];
}
for (int i = 0; i < f1.n * 3; i++)
{
dataGridView1.Rows[i].Cells[0].Value = (i+3)/3;
dataGridView1.Rows[i].Cells[2].Value = D[i];
}
label1.Text = " Перемещения узлов и внешние силы";
break;
case 3:
for (int i = 0; i < f1.n * 3; i++)
{
dataGridView1.Rows[i].Cells[0].Value = D1[i];
}
label1.Text = " Проверка равновесия узлов";
break;
default:
String Str = "Выберите результат вычислений";
//Вывод сообщения
MessageBox.Show(Str, "Ошибка", MessageBoxButtons.OK, MessageBoxIcon.Warning);
return;
}
}
}
}
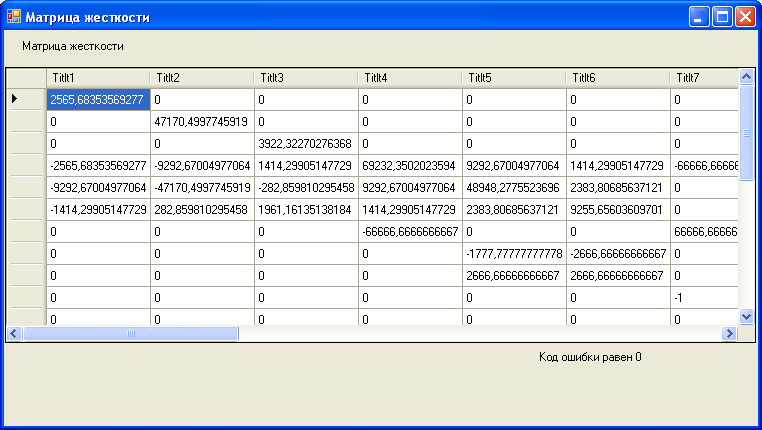
Рис. 6. Форма проекта, представляющая матрицы системы, – форма 2.
Форма3
using System;
using System.Collections.Generic;
using System.ComponentModel;
using System.Data;
using System.Drawing;
using System.Linq;
using System.Text;
using System.Windows.Forms;
using System.Drawing.Drawing2D;
namespace Рама
{
public partial class Form3: Form
{
Form1 f1;
public Form2 f2;
double[,] NQMM;
public Form3(Form1 f1)
{
InitializeComponent();
this.f1 = f1;
f2 = f1.f2;
NQMM=new double[f1.m,5];
NQM();
}
void NQM()
{
if (f2 == null) return;
// массивы косинусов
double[] CA = new double[f1.m];
double[] CB = new double[f1.m];
//dataGridView1.Rows.Add();
//dataGridView1.Rows[0].Cells[0].Value = f2.K[1,1];
for (int ii = 0; ii < f1.m; ii++)
{
NQMM[ii, 0] = ii + 1;
double[] qe = new double[6];
double[] qe1 = new double[6];
double[] u = new double[3];
for (int i=0;i<6;i++)
{
qe1[i]=f1.q[f2.A[ii,i+1]-1];
}
double [,] Ke = new double[3, 3];
// double[,] Ke1 = new double[6, 6];
// double[,] Me = new double[6, 6];
// double[,] Me1 = new double[6, 6];
double[,] T = new double[6, 6];
// double[,] Tt = new double[6, 6];
// double[,] TtK = new double[6, 6];
// double[,] TtM = new double[6, 6];
double x1 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 1]) - 1, 1];
double y1 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 1]) - 1, 2];
double x2 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 2]) - 1, 1];
double y2 = f1.MatrCoor[Convert.ToInt32(f1.MatrTop[ii, 2]) - 1, 2];
double h = Math.Sqrt((x2 - x1) * (x2 - x1) + (y2 - y1) * (y2 - y1));
double cosA = (x2 - x1) / h;
double cosB = (y2 - y1) / h;
double EF = f1.MatrTop[ii, 7];
double EI = f1.MatrTop[ii, 8];
double mm = f1.MatrTop[ii, 9];
CA [ii]=cosA;
CB[ii] = cosB;
//Матрица преобразования координат
T[0, 0] = cosA;
T[0, 1] = cosB;
T[0, 2] = 0;
T[0, 3] = 0;
T[0, 4] = 0;
T[0, 5] = 0;
T[1, 0] = -cosB;
T[1, 1] = cosA;
T[1, 2] = 0;
T[1, 3] = 0;
T[1, 4] = 0;
T[1, 5] = 0;
T[2, 0] = 0;
T[2, 1] = 0;
T[2, 2] = 1;
T[2, 3] = 0;
T[2, 4] = 0;
T[2, 5] = 0;
T[3, 0] = 0;
T[3, 1] = 0;
T[3, 2] = 0;
T[3, 3] = cosA;
T[3, 4] = cosB;
T[3, 5] = 0;
T[4, 0] = 0;
T[4, 1] = 0;
T[4, 2] = 0;
T[4, 3] = -cosB;
T[4, 4] = cosA;
T[4, 5] = 0;
T[5, 0] = 0;
T[5, 1] = 0;
T[5, 2] = 0;
T[5, 3] = 0;
T[5, 4] = 0;
T[5, 5] = 1;
//Локальная матрица жесткости 3*3
Ke[0, 0] = EF / h;
Ke[0, 1] = 0;
Ke[0, 2] = 0;
Ke[1, 0] = 0;
Ke[1, 1] = 12 * EI / Math.Pow(h, 3);
Ke[1, 2] = 6 * EI / Math.Pow(h, 2);
Ke[2, 0] = 0;
Ke[2, 1] = 6 * EI / Math.Pow(h, 2);
Ke[2, 2] = 4 * EI / Math.Pow(h, 1);
// вычисление перемещений в локальной системе отсчета
for (int i = 0; i < 6; i++)
{
qe[i] = 0;
for (int k = 0; k < 6; k++)
qe[i] = qe[i] + T[i, k] * qe1[k];
}
// Вычисление относительных локальных перемещений
double[] deltau = new double[3];
deltau[0]=qe[3]-qe[0];
deltau[1]=qe[5]*h-(qe[4]-qe[1]);
deltau[2]=qe[2]-qe[5];
for (int i = 0; i < 3; i++)
{
NQMM[ii,i+1]=0;
for (int k = 0; k < 3; k++)
NQMM[ii, i + 1] = NQMM[ii, i + 1] + Ke[i, k] * deltau[k];
}
//Изменение знака внутреннего усилия в первом узле
//в соответствие срастяжением нижних волокон при M>0
NQMM[ii, 3] = -NQMM[ii, 3];
dataGridView1.Rows.Add();
//Определение момента во втором узле элемента
NQMM[ii, 4] = NQMM[ii, 3] + NQMM[ii, 2] * h;
for (int i = 0; i < 5; i++)
{
dataGridView1.Rows[ii].Cells[i].Value = NQMM[ii, i];
}
}
//Построение
double[] x, y;
Double My = 0, MaxY = 0, Mx = 0, MaxX = 0;
int[] yint, xint;
Bitmap myBmp;
x = new double[f1.n];
y = new double[f1.n];
for (int i = 0; i < f1.n; i++)
{
x[i] = f1.MatrCoor[i, 1];
y[i] = f1.MatrCoor[i, 2];
}
if (x == null || y == null) return;
MaxY = 0.001;
MaxX = 0.001;
for (int i = 0; i < f1.n; i++)
{
if (System.Math.Abs(y[i]) > MaxY)
{
MaxY = System.Math.Abs(y[i]);;
}
if (System.Math.Abs(x[i]) > MaxX)
{
MaxX = System.Math.Abs(x[i]);
}
}
if (MaxY >= MaxX)
MaxX = MaxY;
else
MaxY = MaxX;
My = (pictureBox1.Height) / 1.2 / MaxY;
Mx = (pictureBox1.Width) / 1.2 / MaxX;
yint = new int[f1.n];
xint = new int[f1.n];
for (int i = 0; i < f1.n; i++)
{
yint[i] = Convert.ToInt32(pictureBox1.Height / 1.1) - Convert.ToInt32(y[i] * My);
xint[i] = Convert.ToInt32(pictureBox1.Width / 10) + Convert.ToInt32(x[i] * Mx);
}
myBmp = new Bitmap(pictureBox1.Width, pictureBox1.Height);
Graphics gr1 = Graphics.FromImage(myBmp);
Pen P1 = new Pen(Color.Red, 2);
gr1.DrawRectangle(P1, 1, 1, pictureBox1.Width - 2, pictureBox1.Height - 2);
Pen P2 = new Pen(Color.Green, 2);
Pen P5 = new Pen(Color.Green, 12);
Brush B2 = new SolidBrush(Color.Azure);
Brush B3 = new SolidBrush(Color.Brown);
int[] Zakx = new int[f1.n];
int[] Zaky = new int[f1.n];
int[] ZakFi = new int[f1.n];
for (int i = 0; i < f1.n; i++)
{
Zakx[i] = Convert.ToInt32(f1.MatrCoor[i, 6]);
Zaky[i] = Convert.ToInt32(f1.MatrCoor[i, 7]);
ZakFi[i] = Convert.ToInt32(f1.MatrCoor[i, 8]);
}
Pen P3 = new Pen(Color.Brown, 4);
Pen P6 = new Pen(Color.Aqua, 18);
for (int i = 0; i < f1.m; i++)
{
//Построение распределенной нагрузки
if ((f1.MatrTop[i, 3]!= 0) || (f1.MatrTop[i, 4]!= 0) || (f1.MatrTop[i, 5]!= 0) || (f1.MatrTop[i, 6]!= 0))
{
gr1.DrawLine(P6, xint[(int)f1.MatrTop[i, 1] - 1], yint[(int)f1.MatrTop[i, 1] - 1], xint[(int)f1.MatrTop[i, 2] - 1], yint[(int)f1.MatrTop[i, 2] - 1]);
}
//Построение линий конечных элементов
gr1.DrawLine(P3, xint[(int)f1.MatrTop[i, 1] - 1], yint[(int)f1.MatrTop[i, 1] - 1], xint[(int)f1.MatrTop[i, 2] - 1], yint[(int)f1.MatrTop[i, 2] - 1]);
}
//Нанесение прямоугольников на расположение узлов
for (int i = 0; i < f1.n; i++)
{
gr1.FillRectangle(B3, xint[i] - 4, yint[i] - 4, 8, 8);
gr1.DrawRectangle(P2, xint[i] - 4, yint[i] - 4, 8, 8);
}
// Линии шириной 12 п. в точках закрепления
for (int i = 0; i < f1.n; i++)
{
if (Zakx[i] < 0 || Zaky[i] < 0)
{
gr1.DrawLine(P5, xint[i] - 24, yint[i] + 10, xint[i] + 24, yint[i] + 10);
}
//Нанесение окружностей на расположение шарниров
if ((Zakx[i]!= 0 || Zaky[i]!= 0) & (ZakFi[i] == 0))
{
gr1.FillEllipse(B2, xint[i] - 6, yint[i] - 6, 12, 12);
gr1.DrawEllipse(P2, xint[i] - 6, yint[i] - 6, 12, 12);
}
}
double Mp = 0, MaxP = 0.001, P = 0;
double[] Px = new double[f1.n];
double[] Py = new double[f1.n];
double[] PM = new double[f1.n];
int[] intPx = new int[f1.n];
int[] intPy = new int[f1.n];
Pen P4 = new Pen(Color.Indigo, 6);
// Стиль пера с наконечниками
P4.SetLineCap(LineCap.Flat, LineCap.ArrowAnchor, DashCap.Flat);
//Изображение сил и моментов
for (int i = 0; i < f1.n; i++)
{
Px[i] = f1.MatrCoor[i, 3];
Py[i] = f1.MatrCoor[i, 4];
PM[i] = f1.MatrCoor[i, 5];
P = Math.Sqrt(Px[i] * Px[i] + Py[i] * Py[i]);
if (MaxP <= P)
MaxP = P;
}
Mp = pictureBox1.Width / 8 / MaxP;
for (int i = 0; i < f1.n; i++)
{
intPy[i] = Convert.ToInt32(Py[i] * Mp);
intPx[i] = Convert.ToInt32(Px[i] * Mp);
}
for (int i = 0; i < f1.n; i++)
{
if (intPx[i]!= 0 || intPy[i]!= 0)
{
gr1.DrawLine(P4, xint[i], yint[i], xint[i] + intPx[i], yint[i] - intPy[i]);
}
if (PM[i] > 0)
{
gr1.DrawArc(P4, xint[i] - 20, yint[i] - 20, 40, 40, 0, -150);
}
if (PM[i] < 0)
{
gr1.DrawArc(P4, xint[i] - 20, yint[i] - 20, 40, 40, 0, 150);
}
}
//Построение эпюры моментов
double[] M1, M2;
M1 = new double[f1.m];
M2 = new double[f1.m];
int[] intM1 = new int[f1.m];
int[] intM2 = new int[f1.m];
double MashtabM = 0, MaxM=0;
for (int i = 0; i < f1.m; i++)
{
M1[i] = NQMM[i, 3];
M2[i] = NQMM[i, 4];
}
for (int i = 0; i < f1.m; i++)
{
if (System.Math.Abs(M1[i]) > MaxM)
{
MaxM = System.Math.Abs(M1[i]);;
}
if (System.Math.Abs(M2[i]) > MaxM)
{
MaxM = System.Math.Abs(M2[i]);
}
}
// 40 пиксклей - максимальное значение на эпюре моментов
MashtabM = 40.0 / MaxM;
for (int i = 0; i < f1.m; i++)
{// Относительная ось моментов направлена вниз, противоположно оси Q
intM1[i] = -Convert.ToInt32(NQMM[i, 3] * MashtabM);
intM2[i] = -Convert.ToInt32(NQMM[i, 4] * MashtabM);
}
Pen P13 = new Pen(Color.Brown, 2);
for (int i = 0; i < f1.m; i++)
{
int xM1=-Convert.ToInt32(intM1[i]*CB[i]);
int xM2 = -Convert.ToInt32(intM2[i] * CB[i]);
int yM1 = -Convert.ToInt32(intM1[i] * CA[i]);
int yM2 = -Convert.ToInt32(intM2[i] * CA[i]);
gr1.DrawLine(P13, xint[(int)f1.MatrTop[i, 1] - 1], yint[(int)f1.MatrTop[i, 1] - 1], xint[(int)f1.MatrTop[i, 1] - 1] +xM1, yint[(int)f1.MatrTop[i, 1] - 1]+yM1);
gr1.DrawLine(P13, xint[(int)f1.MatrTop[i, 2] - 1], yint[(int)f1.MatrTop[i, 2] - 1], xint[(int)f1.MatrTop[i, 2] - 1]+xM2, yint[(int)f1.MatrTop[i, 2] - 1] +yM2);
gr1.DrawLine(P13, xint[(int)f1.MatrTop[i, 1] - 1] + xM1, yint[(int)f1.MatrTop[i, 1] - 1] + yM1, xint[(int)f1.MatrTop[i, 2] - 1] + xM2, yint[(int)f1.MatrTop[i, 2] - 1] + yM2);
}
pictureBox1.Image = myBmp;
}
}
}
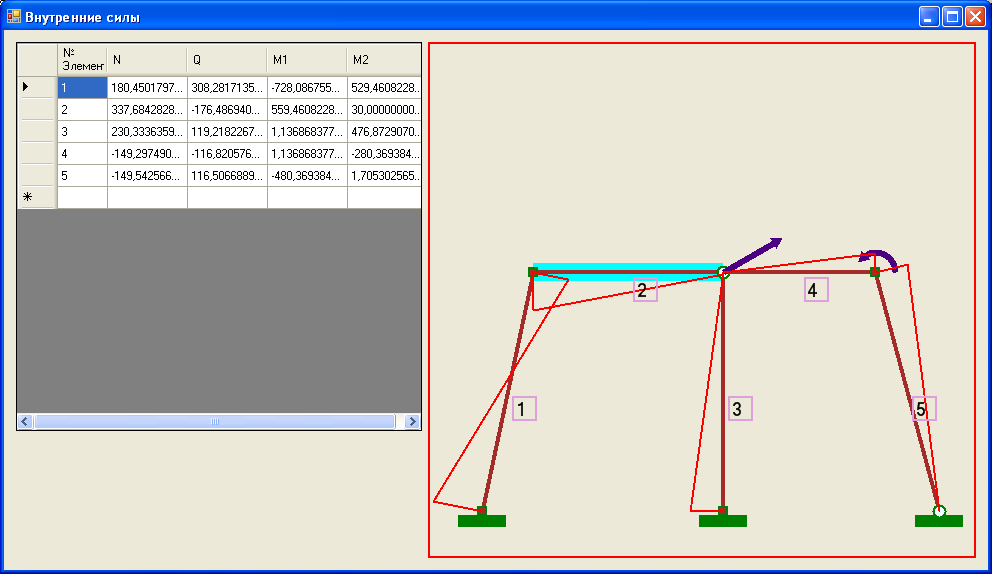
Рис. 7. Форма проекта, отражающая внутренние силовые факторы, – форма 3.
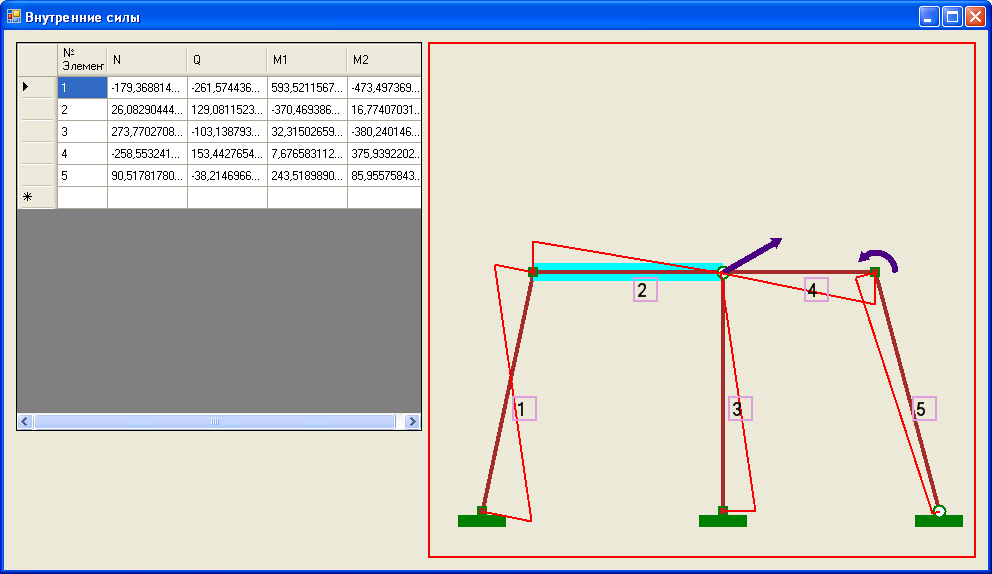
Рис. 8. Форма проекта, отражающая внутренние силовые факторы в динамике при частоте 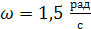 , – форма 3.
, – форма 3.
Варианты заданий:
Определить внутренние силовые факторы в элементах и перемещения узлов плоской рамы в случае статического приложения сил и при заданном гармоническом воздействии при частоте  .
.
Механическая системы, содержащей рамные конечные элементы (рис. 9,10,11), к которой приложены распределенные силы интенсивности p, сосредоточенные сила F и момент M (в статике и в динамике при частоте гармонического воздействия всех сил в одной фазе 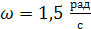 ). Известны размеры
). Известны размеры 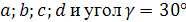 (Таблица 4). Жесткость стержней на растяжение-сжатие составляет EF=
(Таблица 4). Жесткость стержней на растяжение-сжатие составляет EF= 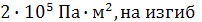 EI=4000
EI=4000 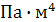 . Удельная масса стержней рамы m=100 кг/м.
. Удельная масса стержней рамы m=100 кг/м.
Таблица 4. Варианты заданий.
| № Варианта | Схема | F, Н | M, Н 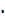 м м
| p, Н/м | a, м | b, м | c, м | d, м |
| рис. 9 | - | |||||||
| рис. 9 | - | |||||||
| рис. 9 | 1,5 | - | ||||||
| рис. 9 | - | |||||||
| рис. 10 | - | |||||||
| рис. 10 | - | |||||||
| рис. 10 | 1,5 | - | ||||||
| рис. 10 | - | |||||||
| рис. 11 | ||||||||
| рис. 11 | ||||||||
| рис. 11 | 1,5 | |||||||
| рис. 11 |
p=40 Н/м, сосредоточенные сила F=700 Н и момент M=200 Н 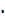 м (в статике и в динамике при частоте гармонического воздействия всех сил в одной фазе
м (в статике и в динамике при частоте гармонического воздействия всех сил в одной фазе 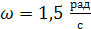 ). Известны размеры
). Известны размеры 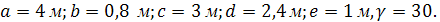
Рис. 9. Варианты 1,2,3,4.
Рис. 10. Варианты 5,6,7,8.
Рис. 11. Варианты 9,10,11,12.